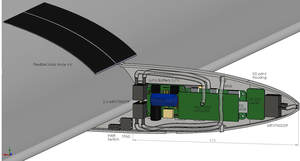
Solar Pilot Guard wins third prize at EAA Air Venture 2021
Our entry was awarded third place at the 2021 EAA Founder’s Innovation Prize to prevent Loss of Control (LOC) at Oshkosh. SPG is a solar charged probe that helps prevent LOC by issuing voice commands over a Bluetooth speaker or cockpit audio. SPG has no moving parts. The wing unit uses 6 ports and 3 accelerations to know dynamic pressures along the longitudinal, vertical and lateral aircraft axes (x, y, z) in combination with a tail unit that can also detect flutter. Python software on the Raspberry Pi Zero is used to identify atmosphere environment effects such as downdrafts, thermals and tipping upsets which can lead to LOC. If LOC is likely, SPG transmits a voice command control response. SPG is like an instructor, customized for the aircraft type. No interfaces to aircraft wire harnesses and existing avionics required.
“SPG is as simple as a stall warning, but is aware of much more.”
|
|
|
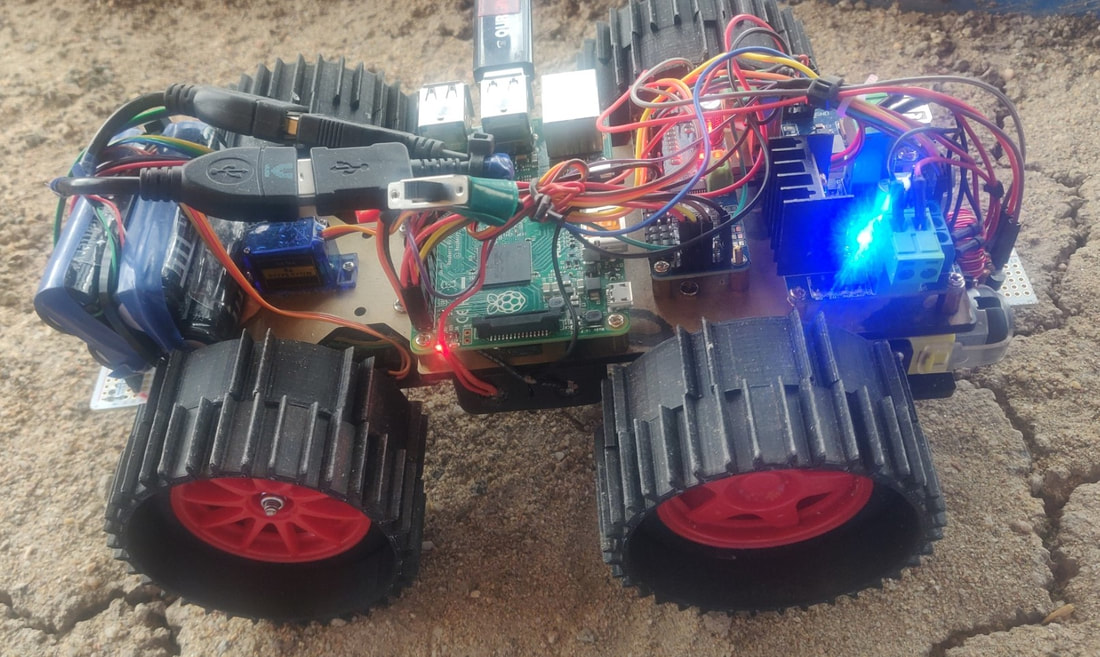
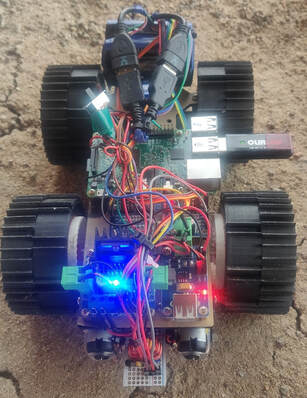
We have a lunar rover analog which uses through-the-wheel sound and seismic audio to characterize the surface and potential hazards of the lunar regolith.
We use machine learning methods to learn the sounds and vibrations of a lunar regolith simulant interacting with the rover wheels. The rover is able to recognize different grades of surface and stop or back up if potentially hazardous fine dust or sand is encountered.
|
|
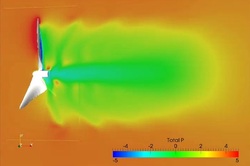
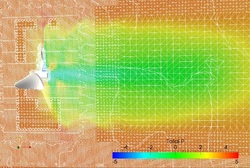
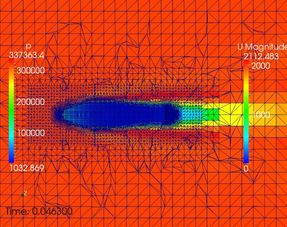
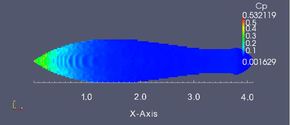
CFD using OpenFoam
Incompressible Flow
Relative Total Pressure (Pa) in the X-Z plane showing the
tip vortex structure, in a 1.5 m/s flow.
|
SnappyHexMesh is good at making a mesh that is more dense near the surface of the object.
|
Velocity Field, streamlines, p and total P.
|
An example of vorticity from the wind turbine.
|
|
|
|
Compressible Flow
Compressible flow - Mach 2
|
Cp over the surface at Mach 2
|
Apollo CSM119
We have worked on a project to repurpose the Apollo Command Module CSM119 to return two people on a journey back from Mars, with assistance from the NASA Johnson Space Center. We were able to reduce the mass of the CSM by roughly 50%, compared to its original weight when manufactured, by taking advantage of reduced mission requirements, current day materials for the heat shield, and up to date avionics. The images below are from a public domain solid model of the Apollo spacecraft (Blender).
Dynamic Rotation of Russian Space Station Mir
Although a flight to Mir is not part of the FAERO business plan, this section illustrates our ability to model physical environments. During the summer of 1997, the Russian space station Mir suffered a number of problems, resulting in the total loss of attitude control. Mike Foale was a crew member at the time, together with Commander Vassily Tsibliev and Flight Engineer Alexander Lazutkin.
A specific inertial attitude is essential for the Mir to keep its solar arrays illuminated by the sun. A rapid energy shortfall, and total loss of power to the station, can result if the arrays are not in a suitable orientation. The usual system of attitude control utilizes sets of momentum wheels, called gyrodynes, spun at high speeds, to provide reactive torques to keep the Mir inertially stable. When an attitude control failure occurs, the angular momentum stored in the gyrodynes is passed into the Mir body as a whole, as the gyrodynes slowly brake their rotation. So even if the Mir looses attitude control in a good orientation with no initial rotation rates, rates build up over the course of hours in all Mir axes, as the gyrodynes spin down.
The immediate problem presented to the crew of Mir, is the establishment of a free body rotation of the Mir to provide sufficient solar illumination of the arrays. With some electrical energy restored, contact with the ground controllers can be established, and workarounds developed to replace the attitude control system box that had failed. The solution developed using the Soyuz transport spacecraft was very crude, but effective. The Soyuz docked to the Mir has a power system independent of the Mir, enabling it to be powered even when the station is not. The Soyuz reaction control jets were manually fired to first arrest the resultant rotation caused by the gyrodines, and then subsequently to reorient Mir, followed finally by a spin of Mir. However, because of the tiny roll moment arm of the Soyuz jets about the Mir long axis, rotation about this axis could not be influenced, complicating the resultant rotation dynamics of Mir.
Much time was spent by the crew considering the effects of initial rotations on the resultant rotation dynamics of Mir. A paper written by Mike Foale was presented to the World Mathematica Conference, Chicago (1998), during which he explained the Euler equations and visualization model that permitted the correct rotation impulse strategy to be applied.
The Mir principal moments of inertial are shown in the graphic below. From the movie it is apparent that although the Mir, with an initial spin about the C axis, is able to present the plane of the solar arrays roughly perpendicular to the sun on the Z axis, the condition does not hold for long. After roughly t≃9 , or about 180 minutes, the A axis is roughly along the Z axis, toward the sun, rotating about the C axis so that the solar arrays cannot properly face the sun. However, this condition is also fairly short lived, and after another 180 minutes the Mir is effectively oriented opposite to the starting condition, but with the A axis again roughly perpendicular to the sun, as can be the solar arrays, if they have rotated their active surface toward the sun.
Analysis of the Euler equations, and their solutions, shows that in general, pure rotations about the principle axis whose moment is the middle value of the three moments A, B, C , (in this case C) are unstable and always result in the behaviour shown above. Pure rotations initiated about axes with the lowest or highest moments of inertia are stable. This suggests that an attempt to spin about the B axis using the Soyuz would result in a stable spin. This is true for Mir, and can be demonstrated using the method above, but unfortunately the solar arrays in that case cannot be oriented continuously with their planes perpendicular to the spin axis, and therefore toward the sun, due to the Mir geometry.
Although clearly not an optimum situation, the rotation described about the C axis was the only realizable option available. Fortunately, it nonetheless provided enough electrical energy for the most essential Mir life support systems to be powered, including communication with the ground. This permitted a stable situation in which repairs could be made, and Mir attitude control could eventually be recovered.
A link to the Mathematica document "Dynamic Rotation of Space Station Mir" is here. It was originally written in Mathematica version 4.0, and subsequently updated to the current version 10.0. This is a single page pdf version of the document "Dynamic Rotation of Space Station Mir.nb".
A specific inertial attitude is essential for the Mir to keep its solar arrays illuminated by the sun. A rapid energy shortfall, and total loss of power to the station, can result if the arrays are not in a suitable orientation. The usual system of attitude control utilizes sets of momentum wheels, called gyrodynes, spun at high speeds, to provide reactive torques to keep the Mir inertially stable. When an attitude control failure occurs, the angular momentum stored in the gyrodynes is passed into the Mir body as a whole, as the gyrodynes slowly brake their rotation. So even if the Mir looses attitude control in a good orientation with no initial rotation rates, rates build up over the course of hours in all Mir axes, as the gyrodynes spin down.
The immediate problem presented to the crew of Mir, is the establishment of a free body rotation of the Mir to provide sufficient solar illumination of the arrays. With some electrical energy restored, contact with the ground controllers can be established, and workarounds developed to replace the attitude control system box that had failed. The solution developed using the Soyuz transport spacecraft was very crude, but effective. The Soyuz docked to the Mir has a power system independent of the Mir, enabling it to be powered even when the station is not. The Soyuz reaction control jets were manually fired to first arrest the resultant rotation caused by the gyrodines, and then subsequently to reorient Mir, followed finally by a spin of Mir. However, because of the tiny roll moment arm of the Soyuz jets about the Mir long axis, rotation about this axis could not be influenced, complicating the resultant rotation dynamics of Mir.
Much time was spent by the crew considering the effects of initial rotations on the resultant rotation dynamics of Mir. A paper written by Mike Foale was presented to the World Mathematica Conference, Chicago (1998), during which he explained the Euler equations and visualization model that permitted the correct rotation impulse strategy to be applied.
The Mir principal moments of inertial are shown in the graphic below. From the movie it is apparent that although the Mir, with an initial spin about the C axis, is able to present the plane of the solar arrays roughly perpendicular to the sun on the Z axis, the condition does not hold for long. After roughly t≃9 , or about 180 minutes, the A axis is roughly along the Z axis, toward the sun, rotating about the C axis so that the solar arrays cannot properly face the sun. However, this condition is also fairly short lived, and after another 180 minutes the Mir is effectively oriented opposite to the starting condition, but with the A axis again roughly perpendicular to the sun, as can be the solar arrays, if they have rotated their active surface toward the sun.
Analysis of the Euler equations, and their solutions, shows that in general, pure rotations about the principle axis whose moment is the middle value of the three moments A, B, C , (in this case C) are unstable and always result in the behaviour shown above. Pure rotations initiated about axes with the lowest or highest moments of inertia are stable. This suggests that an attempt to spin about the B axis using the Soyuz would result in a stable spin. This is true for Mir, and can be demonstrated using the method above, but unfortunately the solar arrays in that case cannot be oriented continuously with their planes perpendicular to the spin axis, and therefore toward the sun, due to the Mir geometry.
Although clearly not an optimum situation, the rotation described about the C axis was the only realizable option available. Fortunately, it nonetheless provided enough electrical energy for the most essential Mir life support systems to be powered, including communication with the ground. This permitted a stable situation in which repairs could be made, and Mir attitude control could eventually be recovered.
A link to the Mathematica document "Dynamic Rotation of Space Station Mir" is here. It was originally written in Mathematica version 4.0, and subsequently updated to the current version 10.0. This is a single page pdf version of the document "Dynamic Rotation of Space Station Mir.nb".
